Definition
A magnetic field is a field that permeates space and which exerts a magnetic force on moving electric charges and magnetic dipoles. Magnetic fields surround electric currents, magnetic dipoles, and changing electric fields.
Basics
See also Magnets
The Magnetic field is the area around a magnet in which a magnetic force is exerted. Moving electric charges produce magnetic fields. Magnetic fields are usually shown by magnetic flux lines. At all times the direction of the magnetic field is shown by the direction of the magnetic flux lines. The strength of a magnet has to do with the spaces between magnetic flux lines. The closer the flux lines are to each other, the stronger the magnet. The farther apart they are, the weaker the magnet. The flux lines can be seen by placing iron filings over a magnet. Magnetic fields give power to other particles that come in contact with the magnetic field. In physics, the magnetic field is a field that permeates space and which exerts a magnetic force on moving electric charges and magnetic dipoles. Magnetic fields surround electric currents, magnetic dipoles, and changing electric fields.
When placed in a magnetic field, magnetic dipoles align their axes to be parallel with the field lines, as can be seen when iron filings are in the presence of a magnet. Magnetic fields also have their own energy and momentum, with an energy density proportional to the square of the field intensity. The magnetic field is measured in the units of teslas (SI units) or gauss (cgs units).
There are some notable specific forms of the magnetic field. For the physics of magnetic materials, see magnetism and magnet, and more specifically ferromagnetism, paramagnetism, and diamagnetism. For constant magnetic fields, such as are generated by stationary dipoles and steady currents, see magnetostatics. For magnetic fields created by changing electric fields, see electromagnetism.
The electric field and the magnetic field are components of the electromagnetic field.
Topics of Interest
Magnetic fields surround magnetic materials and electric currents and are detected by the force they exert on other magnetic materials and moving electric charges. The magnetic field at any given point is specified by both a direction and a magnitude (or strength); as such it is a vector field.
For the physics of magnetic materials, see magnetism and magnet, more specifically ferromagnetism, paramagnetism, and diamagnetism. For constant magnetic fields, such as are generated by magnetic materials and steady currents, see magnetostatics. A changing magnetic field generates an electric field and a changing electric field results in a magnetic field.
In view of special relativity, the electric and magnetic fields are two interrelated aspects of a single object, called the electromagnetic field. A pure electric field in one reference frame is observed as a combination of both an electric field and a magnetic field in a moving reference frame.
In modern physics, the magnetic (and electric) fields are understood to be due to a photon field; in the language of the Standard Model the electromagnetic force is mediated by photons. Most often this microscopic description is not needed because the simpler classical theory covered in this article is sufficient; the difference is negligible under most circumstances.
Definition
In classical physics,the magnetic field 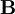 is a vector field (that is, some vector at every point of space and time), with SI units of teslas (one tesla is one newton-second per coulomb-metre) and cgs units of gauss. As a vector field, it has the property of being solenoidal. is a vector field (that is, some vector at every point of space and time), with SI units of teslas (one tesla is one newton-second per coulomb-metre) and cgs units of gauss. As a vector field, it has the property of being solenoidal.
The field 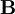 can be both defined and measured by means of a small magnetic dipole (i.e., bar magnet). The magnetic field exerts a torque on magnetic dipoles that tends to make them point in the same direction as the magnetic field (as in a compass),
and moreover the magnitude of that torque is proportional to the
magnitude of the magnetic field. Therefore, in order to measure the
magnetic field at a particular point in space, you can put a small
freely-rotating bar magnet (such as a compass) there: the direction it
winds up pointing is the direction of can be both defined and measured by means of a small magnetic dipole (i.e., bar magnet). The magnetic field exerts a torque on magnetic dipoles that tends to make them point in the same direction as the magnetic field (as in a compass),
and moreover the magnitude of that torque is proportional to the
magnitude of the magnetic field. Therefore, in order to measure the
magnetic field at a particular point in space, you can put a small
freely-rotating bar magnet (such as a compass) there: the direction it
winds up pointing is the direction of 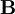 ; and the ratio of the maximum magnitude of the torque to the dipole moment of the bar magnet is the magnitude ; and the ratio of the maximum magnitude of the torque to the dipole moment of the bar magnet is the magnitude 
where ρ is electric charge density. 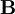 was seen as a kind of magnetic current of vortices aligned in their axial planes, with was seen as a kind of magnetic current of vortices aligned in their axial planes, with 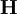 being the circumferential velocity of the vortices. With µ representing
vortex density, we can now see how the product of µ with vorticity
being the circumferential velocity of the vortices. With µ representing
vortex density, we can now see how the product of µ with vorticity 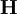 leads to the term magnetic flux density which we denote as leads to the term magnetic flux density which we denote as 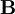 . .
The electric current equation can be viewed as a convective current of electric charge
that involves linear motion. By analogy, the magnetic equation is an
inductive current involving spin. There is no linear motion in the
inductive current along the direction of the 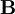 vector. The magnetic inductive current represents lines of force. In
particular, it represents lines of inverse square law force.
vector. The magnetic inductive current represents lines of force. In
particular, it represents lines of inverse square law force.
The extension of the above considerations confirms that where 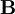 is to is to 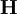 , and where , and where  is to ρ, then it necessarily follows from Gauss's law and from the equation of continuity of charge that is to ρ, then it necessarily follows from Gauss's law and from the equation of continuity of charge that  is to is to  . Ie. . Ie. 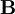 parallels with parallels with  , whereas , whereas 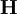 parallels with parallels with 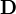 . .
In SI units, 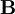 and and 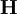 are also related by the equation are also related by the equation
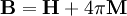 (cgs units), (cgs units),
where 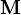 is magnetization. is magnetization.
Visualizing the magnetic field using field lines
Mapping out the strength and direction of the magnetic field is simple in principle. First, measure the strength and direction of the magnetic field at a large number of locations. Then mark each location with an arrow (called a vector) pointing in the direction of the local magnetic field with a length proportional to the strength of the magnetic field. An alternative method of visualizing the magnetic field which greatly simplifies the diagram while containing the same information is to 'connect' the arrows to form "magnetic field lines".
Various physical phenomena have the effect of displaying magnetic field lines. For example, iron filings placed in a magnetic field line up in such a way as to visually show the orientation of the magnetic field. Magnetic fields lines are also visually displayed in polar auroras, in which plasma particle dipole interactions create visible streaks of light that line up with the local direction of Earth's magnetic field.
Field lines provide a simple way to depict or draw the magnetic field (or any other vector field). The magnetic field can be estimated at any point (whether on a field line or not) using the direction and density of the field lines nearby. A higher density of nearby field lines indicates a larger magnetic field.
Field lines are also a good qualitative tool for visualizing magnetic forces. In ferromagnetic substances like iron and in plasmas, magnetic forces can be understood by imagining that the field lines exert a tension, (like a rubber band) along their length, and a pressure perpendicular to their length on neighboring field lines. 'Unlike' poles of magnets attract because they are linked by many field lines; 'like' poles repel because their field lines do not meet, but run parallel, pushing on each other.
The direction of a magnetic field line can be revealed using a compass. A compass placed near the north pole of a magnet points away from that pole—like poles repel. The opposite occurs for a compass placed near a magnet's south pole. The magnetic field points away from a magnet near its north pole and towards a magnet near its south pole. Magnetic field lines outside of a magnet point from the north pole to the south. Not all magnetic fields are describable in terms of poles, though. A straight current-carrying wire, for instance, produces a magnetic field that points neither towards nor away from the wire, but encircles it instead.
B-field lines never end
Field lines are a useful way to represent any vector field and often reveal sophisticated properties of fields quite simply. One important property of the B-field is that it is a solenoidal vector field. In field line terms, this means that magnetic field lines neither start nor end: They always either form closed curves ("loops"), or extend to and from infinity. To date no exception to this rule has been found.
Magnetic field exits a magnet near its north pole and enters near its south pole but inside the magnet B-field lines return from the south pole back to the north. If a B-field line enters a magnet somewhere it has to leave somewhere else; it is not allowed to have an end point. For this reason, magnetic poles always come in N and S pairs. Cutting a magnet in half results in two separate magnets each with both a north and a south pole. Magnetic fields are produced by electric currents, which can be macroscopic currents in wires, or microscopic currents associated with electrons in atomic orbits. The magnetic field B is defined in terms of force on moving charge in the Lorentz force law. The interaction of magnetic field with charge leads to many practical applications. The SI unit for magnetic field is the tesla, which can be seen from the magnetic part of the Lorentz force law Fmag = (qv × B) to be equivalent to (newton × second)/(coulomb × metre). A smaller magnetic field unit is the gauss (1 tesla = 10,000 gauss).
Magnetic monopole (hypothetical)
A magnetic monopole is a hypothetical particle (or class of particles) that has, as its name suggests, only one magnetic pole (either a north pole or a south pole). In other words, it would possess a "magnetic charge" analogous to electric charge.
Modern interest in this concept stems from particle theories, notably Grand Unified Theories and superstring theories, that predict either the existence or the possibility of magnetic monopoles. These theories and others have inspired extensive efforts to search for monopoles. Despite these efforts, no magnetic monopole has been observed to date.
In recent research materials known as spin ices can simulate monopoles, but do not contain actual monopoles.
H-field lines begin and end near magnetic poles
Outside a magnet H-field lines are identical to B-field lines, but inside they point in opposite directions. Whether inside or out of a magnet, H-field lines start near the S pole and end near the N. The H-field, therefore, is analogous to the electric field E which starts as a positive charge and ends at a negative charge. It is tempting, therefore, to model magnets in terms of magnetic charges localized near the poles. Unfortunately, this model is incorrect; it often fails when determining the magnetic field inside of magnets for instance.
The magnetic field and electrical currents
Currents of electrical charges both generate a magnetic field and feel a force due to magnetic B-fields.
All moving charged particles produce magnetic fields. Moving point charges, such as electrons, produce complicated but well known magnetic fields that depend on the charge, velocity, and acceleration of the particles.
Magnetic field lines form in concentric circles around a cylindrical current-carrying conductor, such as a length of wire. The direction of such a magnetic field can be determined by using the "right hand grip rule". The strength of the magnetic field decreases in inverse proportion to the square of the distance from the conductor (inverse-square law).
Bending a current-carrying wire into a loop concentrates the magnetic field inside the loop while weakening it outside. Bending a wire into multiple closely-spaced loops to form a coil or "solenoid" enhances this effect. A device so formed around an iron core may act as an electromagnet, generating a strong, well-controlled magnetic field. An infinitely long electromagnet has a uniform magnetic field inside, and no magnetic field outside. A finite length electromagnet produces essentially the same magnetic field as a uniform permanent magnet of the same shape and size, with its strength and polarity determined by the current flowing through the coil.
The magnetic field generated by a steady current I (a constant flow of charges in which charge is neither accumulating nor depleting at any point) is described by the Biot–Savart law.
Direction of force / the Right hand rule
The direction of force on a positive charge or a current is determined by the right-hand rule. See the figure on the right. Using the right hand and pointing the thumb in the direction of the moving positive charge or positive current and the fingers in the direction of the magnetic field the resulting force on the charge points outwards from the palm. The force on a negatively charged particle is in the opposite direction. If both the speed and the charge are reversed then the direction of the force remains the same. For that reason a magnetic field measurement (by itself) cannot distinguish whether there is a positive charge moving to the right or a negative charge moving to the left. (Both of these cases produce the same current.) On the other hand, a magnetic field combined with an electric field can distinguish between these, see Hall effect below.
An alternative, similar trick to the right hand rule is Fleming's left hand rule.
Earth's magnetic field
Because of Earth's magnetic field, a compass placed anywhere on Earth turns so that the "north pole" of the magnet inside the compass points roughly north, toward Earth's north magnetic pole in northern Canada. This is the traditional definition of the "north pole" of a magnet, although other equivalent definitions are also possible. One confusion that arises from this definition is that if Earth itself is considered as a magnet, the south pole of that magnet would be the one nearer the north magnetic pole, and vice-versa. (Opposite poles attract, so the north pole of the compass magnet is attracted to the south pole of Earth's interior magnet.) The north magnetic pole is so named not because of the polarity of the field there but because of its geographical location.
The figure to the right is a sketch of Earth's magnetic field represented by field lines. For most locations, the magnetic field has a significant up/down component in addition to the North/South component. (There is also an East/West component; Earth's magnetic poles do not coincide exactly with Earth's geological pole.) The magnetic field is as if there were a magnet deep in Earth's interior.
Earth's magnetic field is probably due to a dynamo that produces electric currents in the outer liquid part of its core. Earth's magnetic field is not constant: Its strength and the location of its poles vary. The poles even periodically reverse direction, in a process called geomagnetic reversal.
Source: Wikipedia (All text is available under the terms of the GNU Free Documentation License and Creative Commons Attribution-ShareAlike License.)
|



